Recently, we caught a podcast where a tree was declared 'safe' if it's less than 30% hollow. We think they meant 70% hollow. Either way, this isn't right for several reasons.

We've posted about this before, but as long as this kind of mistake is being broadcast we think it's worth repeating so the message gradually gets home.

The heart of the confusion is the t/R = 0.3 fallacy. t/R = 0.3 is when a residual wall thickness (t) is 30% of the stem radius (R). It's often cited as a failure threshold. It's not. The Why t/R Ratios Don't Work explains why in detail.

In short, one reason is because of a geometric property called section modulus. Wind load and material properties remaining equal, if you double the diameter you increase the load bearing capacity of a tree by 8 times.

To add to the confusion, t/R 0.3 is often referred to as 70% hollow. In fact, a 0.3 t/R ratio is only 50% hollow.  70% is the radius, which is one dimension. t/R 0.3 is the area, which is two dimensions.

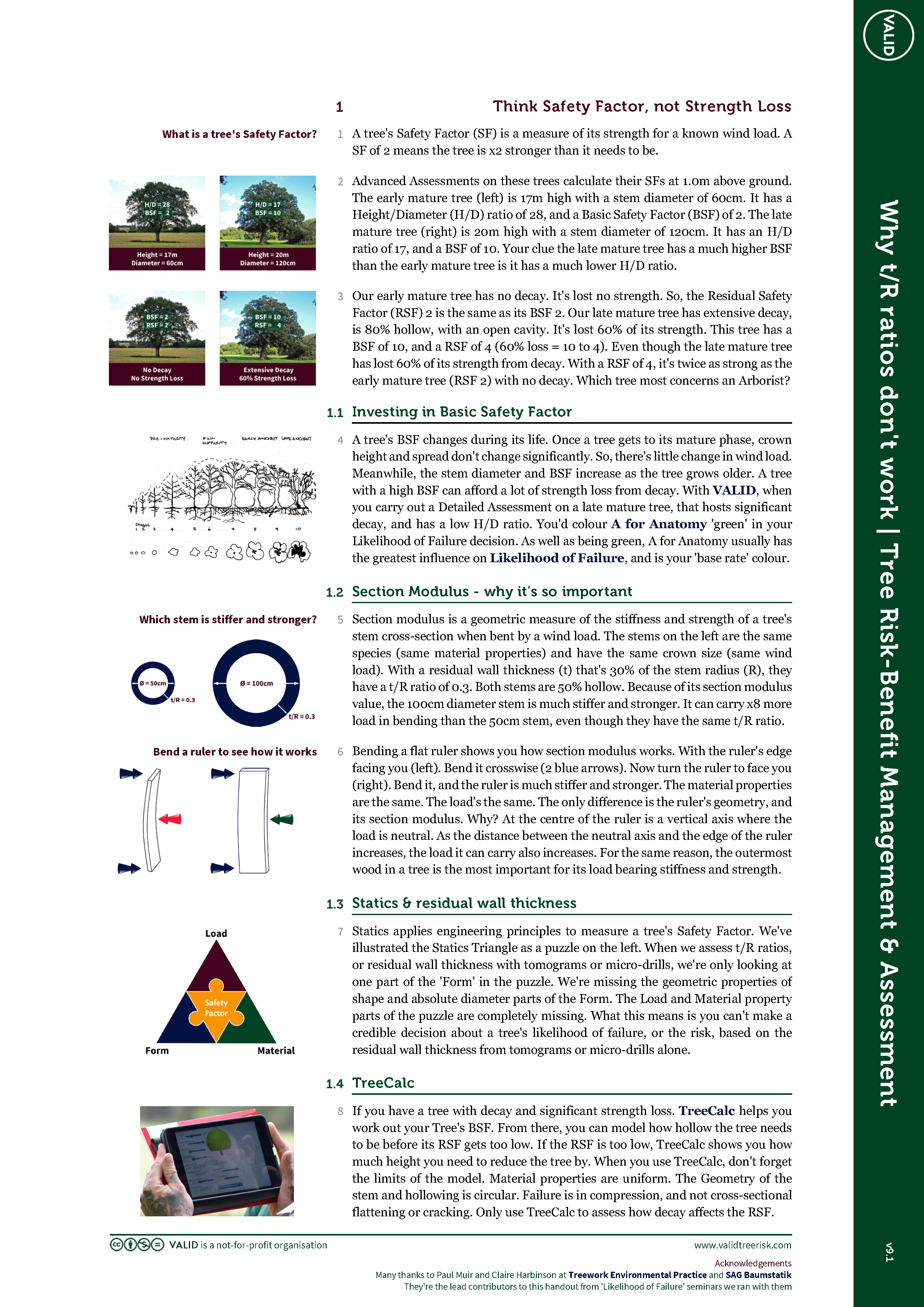
This graph from Paul Muir shows the relationship of central hollowing on:

A = Cross Sectional Area
Z = Section Modulus

t/R = 0.3
A = 49% loss of cross sectional area
Z = 24% reduction in load bearing capacity

To make matters worse. A tree with a t/R ratio of 0.3 can have a very high likelihood of failure, or it can have a very low likelihood of failure.

If all that wasn't enough, it's seldom that where decay is of concern we're dealing with a cross sectional area of a tree that's a circle.